If
you are reading this lesson, then you are pretty far through your geometry
course. The focus of that course has probably concentrated on proving lines
parallel and triangles congruent. In a traditional textbook, this is usually
the first lesson in a unit about quadrilaterals. The two examples of this lesson
model how to classify quadrilaterals with two methods: by all names that apply
or the most precise name that applies. To be able classify quadrilaterals
properly; you must know the definitions of quadrilateral and the special
quadrilaterals.
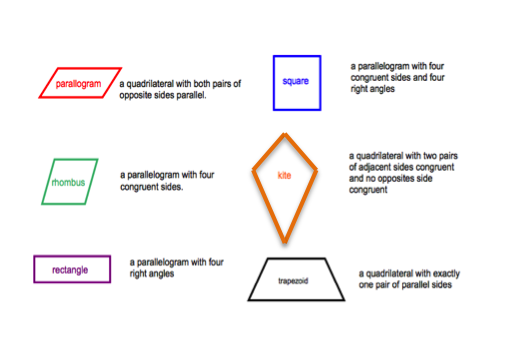
Definitions
Quadrilateral – four-sided figure
Parallelogram – a quadrilateral
with both pairs of opposites sides parallel
Square – a parallelogram with four
congruent sides and four right angles
Rhombus – a parallelogram with four
congruent sides
Kite – a quadrilateral with two
pairs of adjacent sides congruent and no opposite sides congruent
Rectangle – a parallelogram with
four right angles
Trapezoid – a quadrilateral with
exactly one pair of sides parallel
Isosceles Trapezoid – a trapezoid
with the non-parallel sides congruent
Example 1 – Classifying a Quadrilateral
By appearance alone, classify the ABCD in as many ways as
possible.
It is not usual for a person that is studying geometry to be
asked to judge a shape by appearance alone, but in this case, that is what the
directions ask you to do. If you are a verbal learner, then you are probably
capable of just using the definitions to come up with your answer. A visual
learner might find the above image with all the special quadrilaterals paired
with their definitions. No matter how you learn best there are two names for
ABCD:
1.
Quadrilateral
2.
Parallelogram
All too often, students tend to only name it a parallelogram
because it has two pairs of opposite sides parallel. The directions to classify
it in as many ways possible and by definition, it is also can be named a
quadrilateral. It is very important to read and follow the directions to a
problem carefully.
Example 2 – Connecting Algebra and Geometry
Determine the most precise name for the quadrilateral with vertices:
A(-2,1)
B(7,4)
C(4,-1)
D(-2,-3)
The connection between algebra and geometry in this example
is the because the shape is given as vertices on the coordinate plane and to
prove what this shape is relies on two skills: finding the slope of a line and
finding the distance of a line segment. Finding the slope of a line is a skill
taught in the typical Algebra 1 course and finding the length of a line segment
is usually taught early on in a Geometry course.
To help the visual learner, I like to graph the points on
graph paper and create the shape by connecting the vertices. I have done that
in the above image. From the appearance of the shape it appears to be a
trapezoid, because side AB appears to be parallel to side CD. Even though it
appears to be a trapezoid, a proper coordinate proof finds the slope and
distance of every side.
Slopes of Sides
After calculating the slopes, you can see that this is
definitely a trapezoid because sides AB and CD have the same slope and the
other sides do not, thus this quadrilateral has exactly one pair of parallel
sides. Next, I have to prove the lengths of the sides. If sides BC and DA are
the same length, then it would be an isosceles trapezoid, but based on the
graph it does not appear those two sides are the same length.
Lengths of the Sides
Since no side is the same length, this is not an
isosceles trapezoid and the most precise name for this quadrilateral is trapezoid.
Example 3 – Using Properties of Special Quadrilaterals
For the given kite, find the values of the variables and
then find the lengths of the sides.
This example introduces how to use the geometry of special
quadrilaterals to write an equation that needs to be solved. Since the shape is a kite, by definition,
there are two pairs of adjacent sides congruent. From the diagram, it is
apparent that the top two sides are congruent and the bottom two sides are
congruent. Since these sides are congruent we can write equations setting the
algebraic expressions equal to each other:
2x – 12 = 2y – 10
3x – 2 = 2x + 7
To solve this system if equations, I will solve the blue
equation first, because it has only one variable.
(1) 3x –
2 = 2x + 7
(2) x –
2 = 7
(3) x =
9
I change equation (1) into equation (2) by subtracting 2x
from both sides. I change equation (2) into equation (3) by adding 2 to both
sides and the value of x is 9. Now that I know the value of x, I substitute 9
into x in the orange equation.
(1) 2x – 12 = 2y – 10
(2) 2(9) – 12 =
2y – 10
(3) 18 – 12 = 2y
– 10
(4) 6 = 2y – 10
(5) 16 = 2y
(6) y = 8
To solve the orange equation, I first substitute 9 in for x,
which gives equation (2). I simplify 2*9 to give equation (3), then simplify 18
– 12 to give equation (4). To get equation (5), I add 10 to both sides.
Finally, I divide both sides by 2 and use the symmetric property of equality to
turn the equation around to arrive at equation (6) and the second answer to
this problem.
x = 9 and y = 8
To find the lengths of the sides, I substitute 9 for x and 8
for y into the algebraic expressions representing the lengths of the sides of
the kite.
3x – 2 = 3(9) – 2 = 27 – 2 = 25
2x + 7 = 2(9) + 7 = 18 + 7 = 25
2x – 12 = 2(8) – 12 = 18 – 12 = 6
2y – 10 = 2(8) – 10 = 16 – 10 = 6
So, the lengths of the sides are 25, 25, 6, 6 and x = 9,
while y = 8.
As you can see, solving geometry problems can rely heavily
on Algebra skills.
Conclusion – Classifying Quadrilaterals
To be able to classify and solve problems with
quadrilaterals, you need to the definitions of the special quadrilaterals. It
is also important to keep your algebra skills sharp, because many times,
solving problems with quadrilaterals requires solving equations or find the
slope of lines.











 from each side to give
from each side to give












